-
Derivative
The derivative of a function of a real variable measures the sensitivity to change of the function value (output value) with respect to a change in its argument (input value). Derivatives are a fundamental tool of calculus. For example, the derivative of the position of a moving object with respect to time is the object’s velocity: this measures how quickly the position of the object changes when time advances.
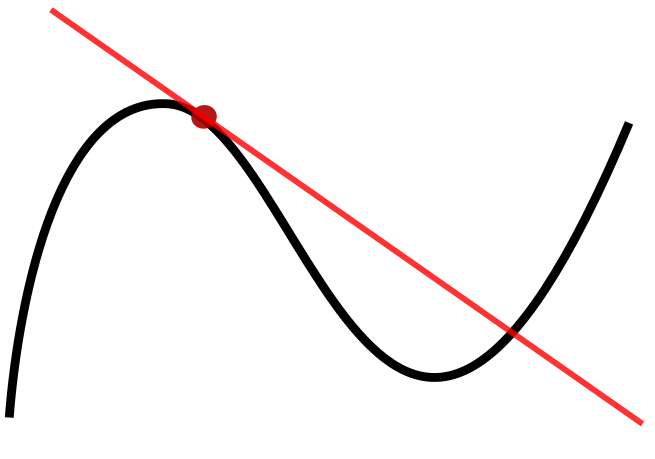
The derivative of a function of a single variable at a chosen input value, when it exists, is the slope of the tangent line to the graph of the function at that point. The tangent line is the best linear approximation of the function near that input value. For this reason, the derivative is often described as the “instantaneous rate of change”, the ratio of the instantaneous change in the dependent variable to that of the independent variable.
Derivatives may be generalized to functions of several real variables. In this generalization, the derivative is reinterpreted as a linear transformation whose graph is (after an appropriate translation) the best linear approximation to the graph of the original function. The Jacobian matrix is the matrix that represents this linear transformation with respect to the basis given by the choice of independent and dependent variables. It can be calculated in terms of the partial derivatives with respect to the independent variables. For a real-valued function of several variables, the Jacobian matrix reduces to the gradient vector.
The process of finding a derivative is called differentiation. The reverse process is called antidifferentiation. The fundamental theorem of calculus relates antidifferentiation with integration. Differentiation and integration constitute the two fundamental operations in single-variable calculus.
-
Derivative (adjective)
Obtained by derivation; not radical, original, or fundamental.
“a derivative conveyance; a derivative word”
-
Derivative (adjective)
Imitative of the work of someone else.
-
Derivative (adjective)
Referring to a work, such as a translation or adaptation, based on another work that may be subject to copyright restrictions.
-
Derivative (adjective)
Having a value that depends on an underlying asset of variable value.
-
Derivative (adjective)
Lacking originality.
-
Derivative (noun)
Something derived.
-
Derivative (noun)
A word that derives from another one.
-
Derivative (noun)
A financial instrument whose value depends on the valuation of an underlying asset; such as a warrant, an option etc.
-
Derivative (noun)
A chemical derived from another.
-
Derivative (noun)
The derived function of a function (the slope at a certain point on some curve f(x))
“The derivative of f:f(x) = x^2 is f’:f'(x) = 2x”
-
Derivative (noun)
The value of this function for a given value of its independent variable.
“The derivative of f(x) = x^2 at x = 3 is f'(3) = 2 * 3 = 6.”
-
Derivate (adjective)
Derived; derivative.
-
Derivate (noun)
Something derived; a derivative.
-
Derivate (verb)
To derive.
