Viscosity vs. Consistency

Main Difference

The main difference between Viscosity and Consistency is that the Viscosity is a physical property of a fluid and Consistency is a in logic, property of a theory that does not contain a contradiction

• Viscosity

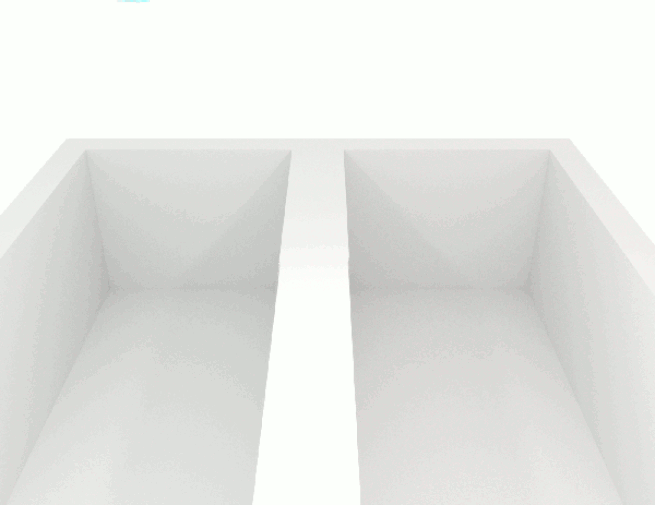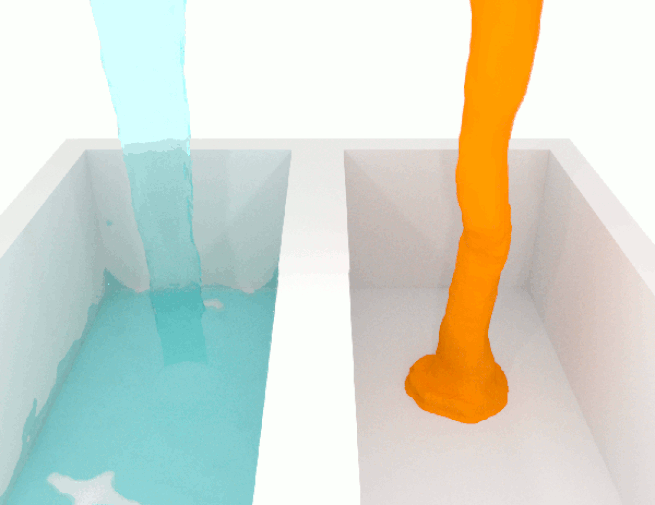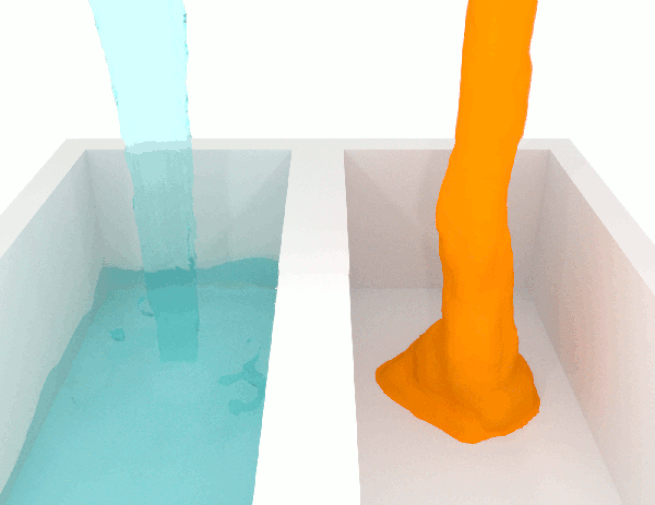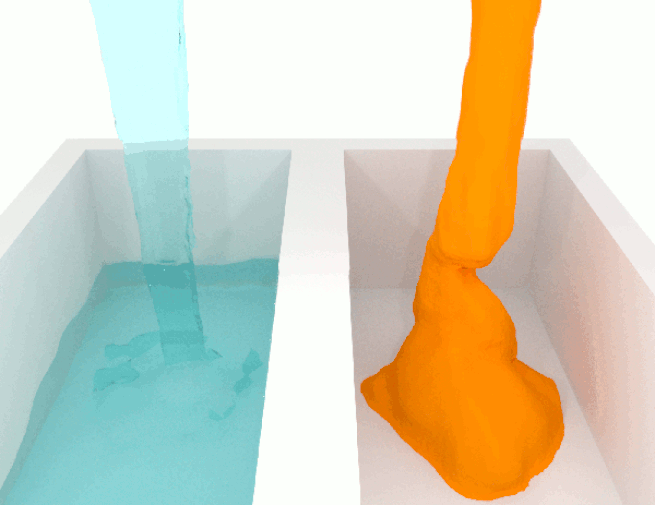
  The viscosity of a fluid is a measure of its resistance to deformation at a given rate. For liquids, it corresponds to the informal concept of “thickness”: for example, syrup has a higher viscosity than water.Viscosity can be conceptualized as quantifying the internal frictional force that arises between adjacent layers of fluid that are in relative motion. For instance, when a fluid is forced through a tube, it flows more quickly near the tube’s axis than near its walls. In such a case, experiments show that some stress (such as a pressure difference between the two ends of the tube) is needed to sustain the flow through the tube. This is because a force is required to overcome the friction between the layers of the fluid which are in relative motion: the strength of this force is proportional to the viscosity.

  A fluid that has no resistance to shear stress is known as an ideal or inviscid fluid. Zero viscosity is observed only at very low temperatures in superfluids. Otherwise, the second law of thermodynamics requires all fluids to have positive viscosity; such fluids are technically said to be viscous or viscid. A fluid with a high viscosity, such as pitch, may appear to be a solid.

• Consistency

  In classical deductive logic, a consistent theory is one that does not entail a contradiction. The lack of contradiction can be defined in either semantic or syntactic terms. The semantic definition states that a theory is consistent if it has a model, i.e., there exists an interpretation under which all formulas in the theory are true. This is the sense used in traditional Aristotelian logic, although in contemporary mathematical logic the term satisfiable is used instead. The syntactic definition states a theory

  T

  {displaystyle T}

  is consistent if there is no formula

  φ

  {displaystyle varphi }

  such that both

  φ

  {displaystyle varphi }

  and its negation

  ¬

  φ

  {displaystyle lnot varphi }

  are elements of the set of consequences of

  T

  {displaystyle T}

  . Let

  A

  {displaystyle A}

  be a set of closed sentences (informally “axioms”) and

  ⟨

  A

  ⟩

  {displaystyle langle Arangle }

  the set of closed sentences provable from

  A

  {displaystyle A}

  under some (specified, possibly implicitly) formal deductive system. The set of axioms

  A

  {displaystyle A}

  is consistent when

  φ

  ,

  ¬

  φ

  ∈

  ⟨

  A

  ⟩

  {displaystyle varphi ,lnot varphi in langle Arangle }

  for no formula

  φ

  {displaystyle varphi }

  .If there exists a deductive system for which these semantic and syntactic definitions are equivalent for any theory formulated in a particular deductive logic, the logic is called complete. The completeness of the sentential calculus was proved by Paul Bernays in 1918 and Emil Post in 1921, while the completeness of predicate calculus was proved by Kurt Gödel in 1930, and consistency proofs for arithmetics restricted with respect to the induction axiom schema were proved by Ackermann (1924), von Neumann (1927) and Herbrand (1931). Stronger logics, such as second-order logic, are not complete.

  A consistency proof is a mathematical proof that a particular theory is consistent. The early development of mathematical proof theory was driven by the desire to provide finitary consistency proofs for all of mathematics as part of Hilbert’s program. Hilbert’s program was strongly impacted by incompleteness theorems, which showed that sufficiently strong proof theories cannot prove their own consistency (provided that they are in fact consistent).

  Although consistency can be proved by means of model theory, it is often done in a purely syntactical way, without any need to reference some model of the logic. The cut-elimination (or equivalently the normalization of the underlying calculus if there is one) implies the consistency of the calculus: since there is no cut-free proof of falsity, there is no contradiction in general.

• Consistency (noun)

  consistent behaviour or treatment

  “the consistency of measurement techniques”

• Consistency (noun)

  the quality of achieving a level of performance which does not vary greatly in quality over time

  “we know we have the ability at the club—what we need is consistency”

• Consistency (noun)

  the way in which a substance holds together; thickness or viscosity

  “the sauce has the consistency of creamed butter”