Theorem vs. Theory

Main Difference

The main difference between Theorem and Theory is that the Theorem is a statement that has been proven on the basis of previously established statements in mathematics and Theory is a contemplative and rational type of abstract or generalizing thinking, or the results of such thinking.

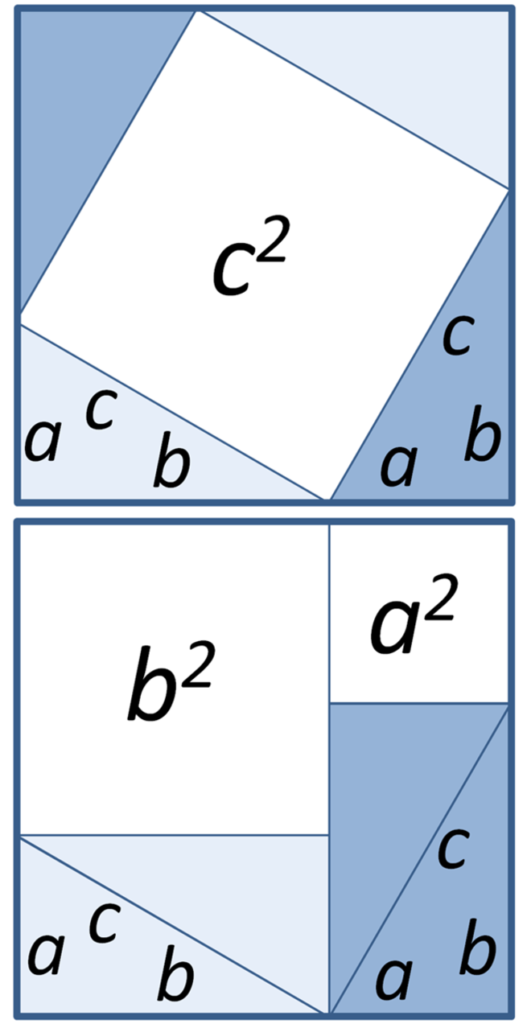
• Theorem

  In mathematics, a theorem is a statement that has been proven on the basis of previously established statements, such as other theorems, and generally accepted statements, such as axioms. A theorem is a logical consequence of the axioms. The proof of a mathematical theorem is a logical argument for the theorem statement given in accord with the rules of a deductive system. The proof of a theorem is often interpreted as justification of the truth of the theorem statement. In light of the requirement that theorems be proved, the concept of a theorem is fundamentally deductive, in contrast to the notion of a scientific law, which is experimental.Many mathematical theorems are conditional statements. In this case, the proof deduces the conclusion from conditions called hypotheses or premises. In light of the interpretation of proof as justification of truth, the conclusion is often viewed as a necessary consequence of the hypotheses, namely, that the conclusion is true in case the hypotheses are true, without any further assumptions. However, the conditional could be interpreted differently in certain deductive systems, depending on the meanings assigned to the derivation rules and the conditional symbol.

  Although they can be written in a completely symbolic form, for example, within the propositional calculus, theorems are often expressed in a natural language such as English. The same is true of proofs, which are often expressed as logically organized and clearly worded informal arguments, intended to convince readers of the truth of the statement of the theorem beyond any doubt, and from which a formal symbolic proof can in principle be constructed. Such arguments are typically easier to check than purely symbolic ones—indeed, many mathematicians would express a preference for a proof that not only demonstrates the validity of a theorem, but also explains in some way why it is obviously true. In some cases, a picture alone may be sufficient to prove a theorem. Because theorems lie at the core of mathematics, they are also central to its aesthetics. Theorems are often described as being “trivial”, or “difficult”, or “deep”, or even “beautiful”. These subjective judgments vary not only from person to person, but also with time: for example, as a proof is simplified or better understood, a theorem that was once difficult may become trivial. On the other hand, a deep theorem may be stated simply, but its proof may involve surprising and subtle connections between disparate areas of mathematics. Fermat’s Last Theorem is a particularly well-known example of such a theorem.

• Theory

  A theory is a contemplative and rational type of abstract or generalizing thinking, or the results of such thinking. Depending on the context, the results might, for example, include generalized explanations of how nature works. The word has its roots in ancient Greek, but in modern use it has taken on several related meanings.

  Theories guide the enterprise of finding facts rather than of reaching goals, and are neutral concerning alternatives among values. A theory can be a body of knowledge, which may or may not be associated with particular explanatory models. To theorize is to develop this body of knowledge.

  As already in Aristotle’s definitions, theory is very often contrasted to “practice” (from Greek praxis, πρᾶξις) a Greek term for doing, which is opposed to theory because pure theory involves no doing apart from itself. A classical example of the distinction between “theoretical” and “practical” uses the discipline of medicine: medical theory involves trying to understand the causes and nature of health and sickness, while the practical side of medicine is trying to make people healthy. These two things are related but can be independent, because it is possible to research health and sickness without curing specific patients, and it is possible to cure a patient without knowing how the cure worked.

  In modern science, the term “theory” refers to scientific theories, a well-confirmed type of explanation of nature, made in a way consistent with scientific method, and fulfilling the criteria required by modern science. Such theories are described in such a way that any scientist in the field is in a position to understand and either provide empirical support (“verify”) or empirically contradict (“falsify”) it. Scientific theories are the most reliable, rigorous, and comprehensive form of scientific knowledge, in contrast to more common uses of the word “theory” that imply that something is unproven or speculative (which is better characterized by the word hypothesis). Scientific theories are distinguished from hypotheses, which are individual empirically testable conjectures, and from scientific laws, which are descriptive accounts of how nature behaves under certain conditions.